Great geophysicists #11: Thomas Young
/ Painting of Young by Sir Thomas LawrenceThomas Young was a British scientist, one of the great polymaths of the early 19th century, and one of the greatest scientists. One author has called him 'the last man who knew everything'¹. He was born in Somerset, England, on 13 June 1773, and died in London on 10 May 1829, at the age of only 55.
Painting of Young by Sir Thomas LawrenceThomas Young was a British scientist, one of the great polymaths of the early 19th century, and one of the greatest scientists. One author has called him 'the last man who knew everything'¹. He was born in Somerset, England, on 13 June 1773, and died in London on 10 May 1829, at the age of only 55.
Like his contemporary Joseph Fourier, Young was an early Egyptologist. With Jean-François Champollion he is credited with deciphering the Rosetta Stone, a famous lump of granodiorite. This is not very surprising considering that at the age of 14, Young knew Greek, Latin, French, Italian, Hebrew, Chaldean, Syriac, Samaritan, Arabic, Persian, Turkish and Amharic. And English, presumably.
But we don't include Young in our list because of hieroglyphics. Nor because he proved, by demonstrating diffraction and interference, that light is a wave — and a transverse wave at that. Nor because he wasn't a demented sociopath like Newton. No, he's here because of his modulus.
Elasticity is the most fundamental principle of material science. First explored by Hooke, but largely ignored by the mathematically inclined French theorists of the day, Young took the next important steps in this more practical domain. Using an empirical approach, he discovered that when a body is put under pressure, the amount of deformation it experiences is proportional to a constant for that particular material — what we now call Young's modulus, or E:
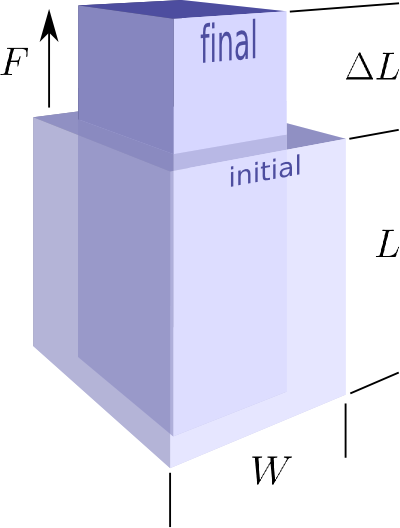 This well-known quantity is one of the stars of the new geophysical pursuit of predicting brittleness from seismic data, and a renewed interested in geomechanics in general. We know that Young's modulus on its own is not enough information, because the mechanics of failure (as opposed to deformation) are highly nonlinear, but Young's disciplined approach to scientific understanding is the best model for figuring it out.
This well-known quantity is one of the stars of the new geophysical pursuit of predicting brittleness from seismic data, and a renewed interested in geomechanics in general. We know that Young's modulus on its own is not enough information, because the mechanics of failure (as opposed to deformation) are highly nonlinear, but Young's disciplined approach to scientific understanding is the best model for figuring it out.
Sources and bibliography
- The Last Man Who Knew Everything by Andrew Robinson
- A great blog post about that book
- Wikipedia has a thorough review of Young's life and work, natch.
- A very nice audio essay written by Paul Riddel, and published by ABC.
- There's quite a bit on Young in Simon Singh's brilliant The Code Book, which I highly recommend.
Footnote
¹ Thomas Young wrote a lot of entries in the 1818 edition of Encyclopædia Britannica, including pieces on bridges, colour, double refraction, Egypt, friction, hieroglyphics, hydraulics, languages, ships, sound, tides, and waves. Considering that lots of Wikipedia is from the out-of-copyright Encyclopædia Britannica 11th ed. (1911), I wonder if some of Wikipedia was written by the great polymath? I hope so.
Just found out it's also James Clerk Maxwell's birthday today — he's on our list too. Maybe we'll get to him next June...










 Except where noted, this content is licensed
Except where noted, this content is licensed