Are conferences failing you too?
/I recently asked a big software company executive if big exhibitions are good marketing value. The reply:
It's not a waste of money. It's a colossal waste of money.
So that's a 'no'.
Is there a problem here?
Next week I'll be at the biggest exhibition (and conference) in our sector: the SEG Annual Meeting. Thousands of others will be there, but far more won’t. Clearly it’s not indispensable or unmissable. Indeed, it’s patently missable — I did just fine in my career as a geophysicist without ever going. Last year was my first time.
Is this just the nature of mass market conferences? Is the traditional academic format necessarily unremarkable? Do the technical societies try too hard to be all things to all people, and thereby miss the mark for everyone?
I don't know the answer to any of these questions, I can only speak for myself. I'm getting tired of conferences. Perhaps I've reached some new loop in the meandering of my career, or perhaps I'm just grumpy. But as I've started to whine, I'm finding more and more allies in my conviction that conferences aren't awesome.
What are conferences for?
- They make lots of money for the technical societies that organize them.
- A good way to do this is to provide marketing and sales opportunities for the exhibiting vendors.
- A good way to do this is to attract lots of scientists there, baiting with talks by all the awesomest ones.
- A good way to do this, apparently, is to hold it in Las Vegas.
But I don't think the conference format is great at any of these things, except possibly the first one. The vendors get prospects (that's what sales folk call people) that are only interested in toys and beer — they might be users, but they aren't really customers. The talks are samey and mostly not memorable (and you can only see 5% of them). Even the socializing is limited by the fact that the conference is gigantic and run on a tight schedule. And don't get me started on Las Vegas.
 If we're going to take the trouble of flying 8000 people to Las Vegas, we had better have something remarkable to show for it. Do we? What do we get from this giant conference? By my conservative back-of-the-envelope calculation, we will burn through about 210 person-years of productivity in Las Vegas next week. That's about 6 careers' worth. Six! Are we as a community satisfied that we will produce 6 careers' worth of insight, creativity, and benefit?
If we're going to take the trouble of flying 8000 people to Las Vegas, we had better have something remarkable to show for it. Do we? What do we get from this giant conference? By my conservative back-of-the-envelope calculation, we will burn through about 210 person-years of productivity in Las Vegas next week. That's about 6 careers' worth. Six! Are we as a community satisfied that we will produce 6 careers' worth of insight, creativity, and benefit?
You can probably tell that I am not convinced. Tomorrow, I will put away the wrecking ball of bellyaching, and offer some constructive ideas, and a promise. Meanwhile, if you have been to an amazing conference, or can describe one from your imagination, or think I'm just being a grouch — please use the comments below.
Map data ©2012 Google, INEGI, MapLink, Tele Atlas.
Don't miss the next post, Ways to experiment with conferences.






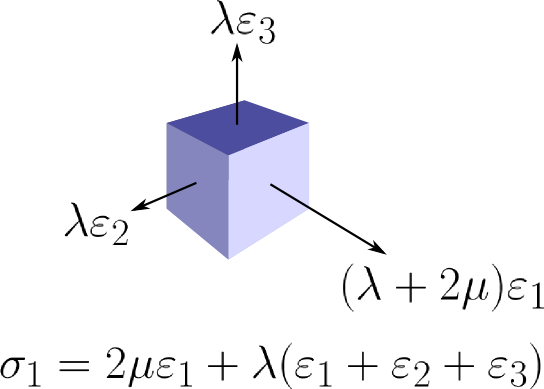
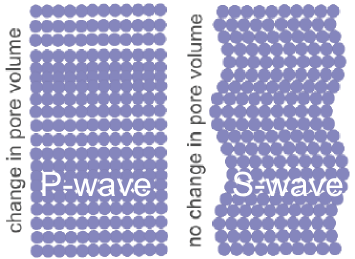
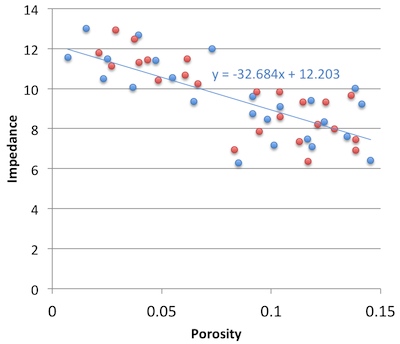
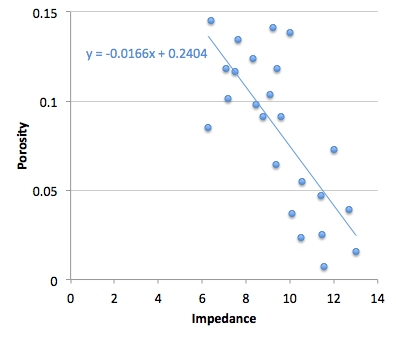









 Except where noted, this content is licensed
Except where noted, this content is licensed